Present ValuePV Defined & Formula
The value of your money today is worth more than its value in the future.
Do you think that receiving $1,000 in the future is the same or even better than receiving it now?
Okay, let us put it this way.
Imagine how much stuff you can buy with $500 about five years ago.
Compare that to now.
Which $500 do you think had more value?
The $500 of five years ago, or the $500 of today?
Business owners (whether consciously or unconsciously) deal with the concepts of present and future values in their day-to-day business operations.
The interest expense on loans, the returns from investments, the value of properties today compare to their values in the future, accounting for inflation – all of these are within the scope of present and future values.

What is Present Value?
It’s easy to choose between the $500 of today and the $500 in the future.
Most people would take the $500 of today and not think twice.
But what if it’s a choice between $500 today or $550 five years in the future?
That’s where the concept of present value comes in.
Present value refers to the current value of money that you expect to receive in the future.
In other words, it is the value of the money you would receive in the future if you were to receive it today instead.
But how is this done?
The usual method is to discount the future value of money at the discount rate.
It could be the interest rate, inflation rate, or any other factors that can affect the future value of money.
Prevent value (PV) can be computed using the formula:
Present Value = Future Value ÷ (1+r)n
Where:
r = discount rate
n = the number of periods (month, quarter, year, etc.)
Let’s go back to picking between $500 today or $550 five years in the future.
Before we can decide which to take, we need to consider the present value of the $550.
For simplicity, let’s just say that the discount rate is the interest rate if we were to put the money in the bank, and it is 3%.
Let’s compute for the present value of the $550 that we could potentially receive five years from now.
Present Value = Future Value ÷ (1+r)n
Present Value = $550 ÷ (1 + .03)5
Present Value =$550 ÷ 1.1593
Present Value = $474.43
So assuming that it would have an interest rate of 3%, then the present value of the $550 is $474.43, which is less than the $500 that we can receive today.
In this case, it’s more beneficial to choose the $500.
What if instead of receiving $550 after five years, we receive $600?
Would it still be beneficial to go with the $500 choice?
Assuming the discount rate is still the same, let’s compute for the present value of the $600:
Present Value = Future Value ÷ (1+r)n
Present Value = $600 ÷ (1 + .03)5
Present Value =$600 ÷ 1.1593
Present Value = $517.57
So now, the present value is $517.57 which is more than the $500 we can receive today.
In this case, it’s more beneficial to go with the $600 even if we have to wait for five years.
What is Future Value?
So while we were discussing the concept of present value, “future value” was mentioned a number of times.
It is even included in the computation for present value. So it’s only appropriate to discuss what future value is.
Future value is the expected value of present money after a certain amount of time has passed.
For example, if you were to put your money in a bank knowing that it would earn interest, the money you put plus the interest it earns will be its future value.
From the present value formula, we can derive the formula for future value:
Future Value = Present Value x (1+r)n
Where:
r = interest rate
n= number of periods
Let’s go back to our $500 vs $550/$600 example earlier. This time, we’ll compute for the future value of the $500:
Future Value = Present Value x (1+r)n
Future Value = $500 x (1+.03) 5
future Value = $500 x 1.1593
Future Value = $579.64
So if we were to receive the $500 and put it in a bank that offers 3% annually, then after five years, we will have $579.64.
What is the discount rate and how do I determine it?
As shown in our present value formula above, a discount rate is the rate of return or interest rate that we use to discount future cash flows so that we can determine their present value.
Determining what discount rate to use is fairly subjective.
It’ll depend on the business.
It could be the perceived rate of return of an investment, the interest rate if the money was deposited in a bank instead, a pre-defined hurdle rate, etc.
If you want the most conservative computation, you can refer to a risk-free rate of return, which is usually the interest rate of treasury bonds.
Since treasury bonds are backed by the government, it is generally risk-free and you can be assured that you’ll get paid the amount promised by the bond.
This is why it’s a good basis if you want to be conservative with your computation.
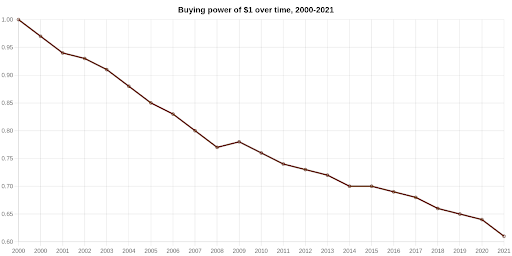
Inflation and Purchasing Power
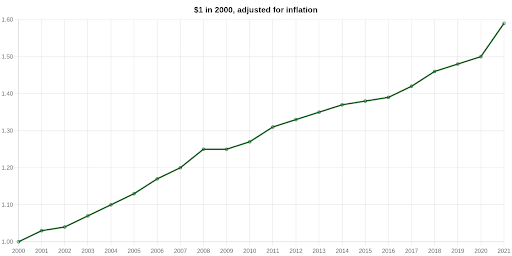
Source: https://www.officialdata.org/us/inflation/2000?amount=1
There’s a reason as to why a dollar today is worth more than a dollar a year from now.
The prices of commodities go up, stuff becomes expensive over time… a dollar’s value just decreases over time.
Let’s take the McDonald’s Big Mac meal for example.
In 2000, a Big Mac meal will only cost you $2.39.
Fast forward to 2021, a Big Mac meal will cost you $5.99.
That’s more than a $2 difference!
The dollar of 2000 can buy more stuff than the dollar of 2021.
Inflation measures the rate of how much the prices of goods and services rise over time.
Historically, the prices of goods and services have been continually going up.
The increase in prices could be caused by an increase in the supply of money, an increase in demand, scarcity of supply, etc.
But whatever the cause is, if there is inflation, the amount of stuff that a dollar can buy (referred to as purchasing power) goes down.
Any unspent money that does not earn income will eventually decrease in value.
It could be due to inflation. It could be due to a potential return if it was invested.
Sure, a $1 bill may still be a $1 bill after a year or five years, but the amount of stuff that it can buy won’t be the same.
It would most probably be less.
Use of Present Value in Business
The concept of present value is useful for a business.
It can help in investment decisions, such as assessing whether it’s worth it to invest in a particular equity instrument or not, or whether it’s worth it to purchase a piece of machinery to increase operational capacity for an opportunity for increased sales.
The business will take into account the present value of the returns in investment, the opportunity cost should the money have been utilized in other income-generating sources, the interest rate, etc.
For example, company LJ has been presented with an investment opportunity that will earn $15,000/year.
Company LJ would have to invest $50,000 in order to avail of such an opportunity.
Coincidentally, a time deposit of $50,000 just matured and is now available for reinvestment.
Company LJ has the option to renew the time deposit for another five years, earning 10% interest per year.
The company’s management is now wondering if it’s worth it to take the investment opportunity, or if it’s better to just renew the time deposit.
Let’s compute first for the present value of the investment opportunity.
For the discount rate, we will use the opportunity cost of 10% which is what the $50,000 would earn if the time deposit was renewed.
The formula for computing the present value for multiple inflows is:
| Present Value = | FV | + | FV | + | FV | + | FV | + | ….. |
| (1+r)^n | (1+r)^n | (1+r)^n | (1+r)^n |
Where:
FV = Future Value
r = Discount rate
n = number of period (e.g. for year 1, n=, for year 2, n=2…)
Using this formula, let’s compute the present value of the investment opportunity:
| Present Value = | FV | + | FV | + | FV | + | FV | + | FV |
| (1+r)^n | (1+r)^n | (1+r)^n | (1+r)^n | (1+r)^n |
| Present Value = | 15,000.00 | + | 15,000.00 | + | 15,000.00 | + | 15,000.00 | + | 15,000.00 |
| (1+.10)^1 | (1+.10)^2 | (1+.10)^3 | (1+.10)^4 | (1+.10)^5 |
Present Value = 13,636.36 + 12,396.69 + 11,269.72 + 10,245.20 + 9,318.82
Present Value = 56,861.80
We can also present the computation via table:
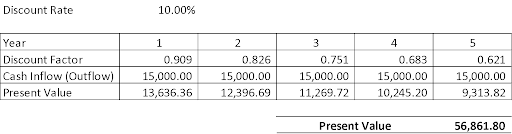
*Discount Factor = 1 ÷ (1+r)n
So as per our computation, the present value of the investment opportunity is $56,861.80.
This more than makes up for the $50,000 that company LJ needs to invest, so it could be beneficial for them if they take the investment opportunity.
Let’s have another example.
A gaming arcade is planning to purchase a vending machine that costs $10,000.
This vending machine has an expected useful life of five years and has a salvage value of $1,500.
It is expected to earn $3,000/year during its useful life.
After five years, the gaming arcade will sell it for its salvage value.
Alternatively, the gaming arcade can put the $10,000 as a time deposit, earning 12% annually.
Let’s compute the present value of the vending machine to see if it’s a worthy investment:
| Present Value = | FV | + | FV | + | FV | + | FV | + | FV | ||||||||||||||||||
| (1+r)^n | (1+r)^n | (1+r)^n | (1+r)^n | (1+r)^n | |||||||||||||||||||||||
| Present Value = | 3,000.00 | + | 3,000.00 | + | 3,000.00 | + | 3,000.00 | + | 3,000+ 1,500 | ||||||||||||||||||
| (1+.12)^1 | (1+.12)^2 | (1+.12)^3 | (1+.12)^4 | (1+.12)^5 | |||||||||||||||||||||||
| Present Value = | 3,000.00 | + | 3,000.00 | + | 3,000.00 | + | 3,000.00 | + | 4,500.00 | ||||||||||||||||||
| 1.12 | 1.2544 | 1.4049 | 1.5735 | 1.7623 | |||||||||||||||||||||||
Present Value = 2,678.57 + 2,391.58 + 2,135.34 + 1,906.55 + 2,553.42
Present Value = 11,665.47
And presenting the computation in table form:
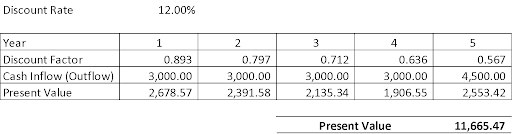
As per our computation, the present value of the cash inflows from the vending machine is $11,665.
It is more than the acquisition cost of $10,000 which makes it a worthy investment.
The gaming arcade will probably benefit from the purchase of the vending machine.
Limitations of Present Value
The main limitation of computing present value is the subjectivity of determining the discount rate.
Since there is no one standard for the discount rate, there’s a chance that the computed present value could be inaccurate.
For example, if the discount rate we used is 10% but it turned out that the actual rate of return is 8%.
The actual present value and the computed present value will be unequal.
Another limitation of computing present value is that we make a lot of assumptions – the future cash inflows, outflows, the discount rate.
Since it is all assumptions about the future, the calculated present value is probably not 100% accurate.
Interest rates might change, unexpected expenditures might be incurred, the rate of return might fluctuate… these things cannot be included in the computation of present value.
But even with these limitations, computing the present value of your money or potential investments is still beneficial for your business.
FundsNet requires Contributors, Writers and Authors to use Primary Sources to source and cite their work. These Sources include White Papers, Government Information & Data, Original Reporting and Interviews from Industry Experts. Reputable Publishers are also sourced and cited where appropriate. Learn more about the standards we follow in producing Accurate, Unbiased and Researched Content in our editorial policy.
Easter Illinois University "Financial Functions Using Microsoft Excel" Page 1. September 28, 2021
Arkansas Tech University "Present and Future Value of a Continuous Income Stream" Page 1. September 28, 2021
Illinois Tech "NPV calculation" Page 1 . September 28, 2021
Officialdata.org "$1 in 2000 is worth $1.59 today " Page 1. September 28, 2021